Abstract:
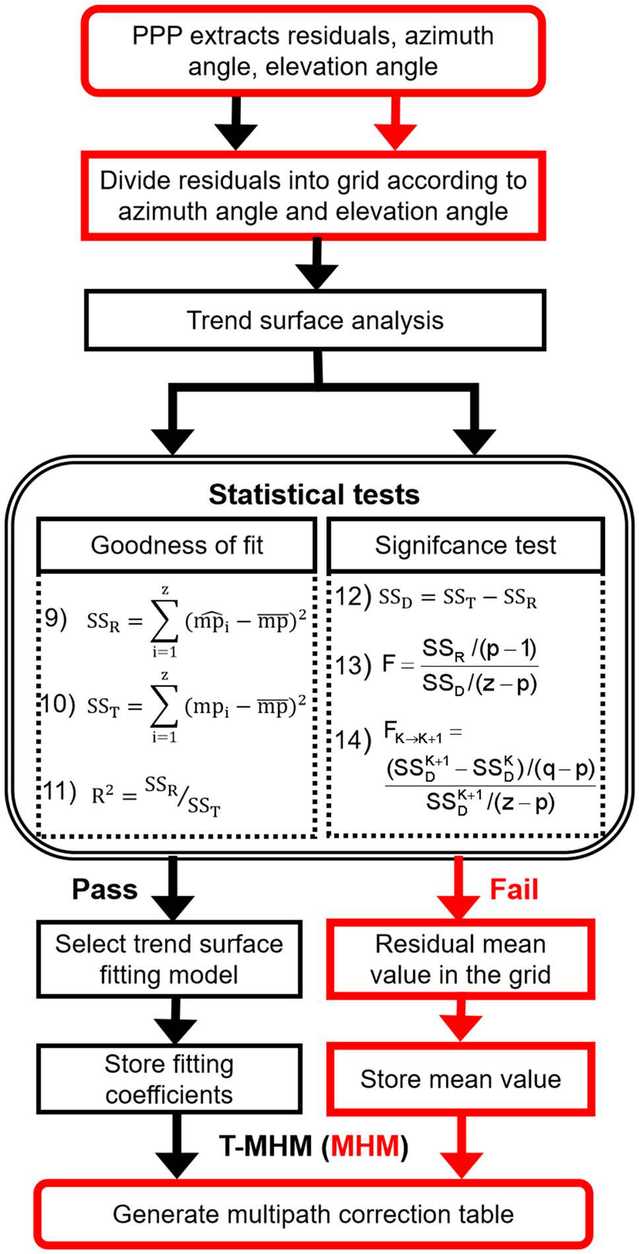
The multipath effect reduces the accuracy of pseudorange and carrier phase observations and significantly affects the convergence of precise point positioning (PPP) in an urban high-obstruction environment. The multipath hemispherical map (MHM) is based on the spatial repeatability of the multipath and is used to mitigate the multipath effect. This method is widely used because it is suitable for real-time dynamic and static situations with multipath invariance. Although the MHM algorithm is simple and easy to implement, it ignores the spatial distribution of multipath inside the sky grid. It is not suitable for use in high-frequency multipath corrections. By fitting the multipath trend inside the sky grid, the MHM based on a trend-surface analysis (T-MHM) alleviates the high-frequency and low-frequency multipath simultaneously, improving the accuracy of the baseline solution in the differential mode. We mainly demonstrate the application of T-MHM in PPP, analyze the unmodeled errors, evaluate the multipath correction effect of T-MHM, discuss the optimal modeling days, and test its sensitivity in the scale of the sky grid. Compared to MHM, the experimental results show that using T-MHM in multipath correction improves the positioning accuracy in the horizontal and vertical directions by 21.6 and 13.6%, respectively, and shortens the convergence time by 24.2 and 7.2%, respectively. The T-MHM method is not sensitive to the grid scale of the sky, thus resulting in high efficiency.
全文链接: